View larger image

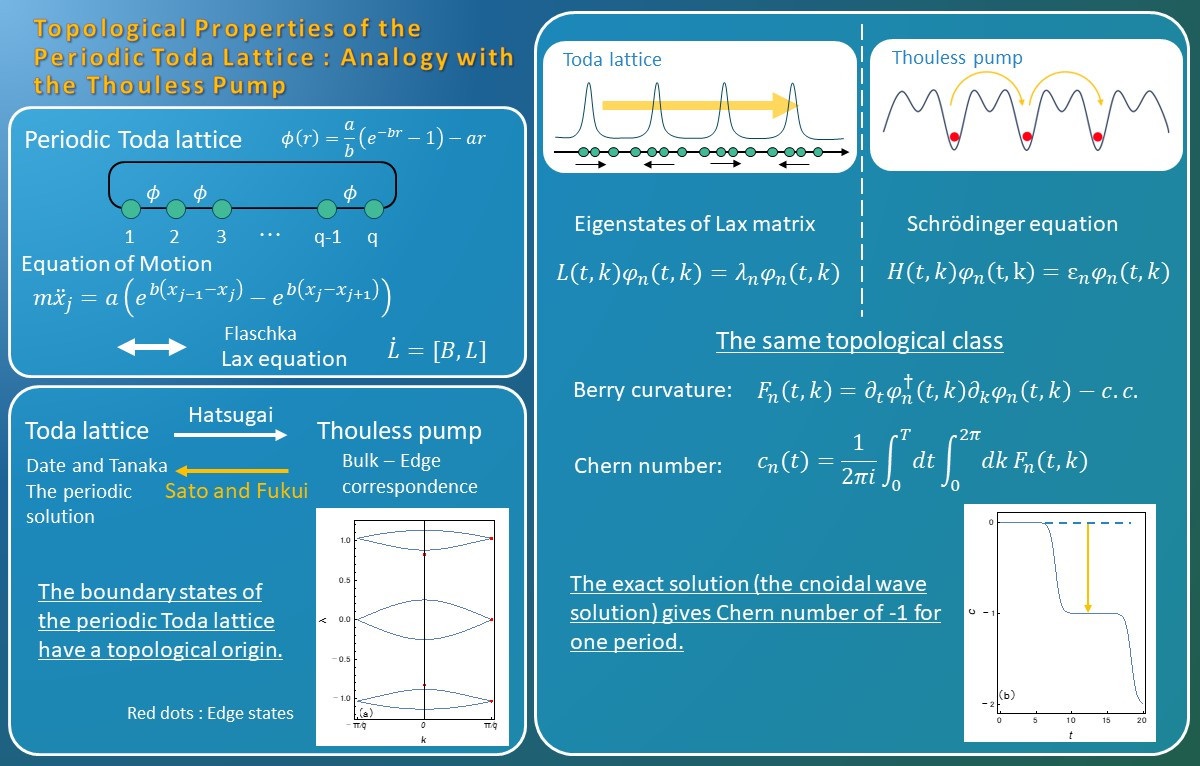
The Toda lattice discovered by Morikazu Toda in 1967 is a one-dimensional lattice model with an exponential-type interaction. Despite the nonlinear interaction, the Toda lattice is completely integrable. The Lax equation introduced by Flaschka in 1974, which is a matrix format of the canonical equations of motion, has led to the proof of the integrability. In 1976, Date and Tanaka obtained generic periodic solutions of the Toda lattice using the eigenvalues of the Lax matrix.

On the other hand, the Thouless pump introduced by Thouless in 1983 is a quantum pump that transports particles in periodically and adiabatically moving potentials. The Thouless pump is often called the topological pump, because the number of transported particles in one period is quantized, given by a topological invariant known as the Chern number.

In our study, we shed light on the topological properties of the periodic Toda lattice. At first glance, the Toda lattice appears to be unrelated to the Thouless pump. However, in 1993, Hatsugai has established the theory of the bulk-edge correspondence (BEC) in topological phenomena in condensed matter physics, based on the techniques developed by Date and Tanaka. Conversely, in the study, we have reconsidered the Lax eigenvalue equation of the Toda lattice from the viewpoint of the BEC. Namely, we have first confirmed that the boundary states that play a crucial role in obtaining the exact solutions of the Toda lattice, have a topological origin. In the spirit of the BEC, we have subsequently shown that the bulk eigenfunctions of the Lax matrix on the periodic Toda lattice yield nontrivial Chern numbers. In particular, the cnoidal wave solution derived by Toda exhibits a Chern number of -1. This implies that the periodic Toda lattice belongs to the same topological class as the Thouless pump.

This result shows that a topological viewpoint can be useful in the study of solvable models of nonlinear waves. As is widely known, solitons are not topologically protected but are due to the balance between nonlinearity and dispersion in the wave propagation. Our result suggests that the topological properties of the Toda lattice may be involved in the stability of solitons. Investigating whether other solvable models of nonlinear waves have the same topological properties will be noteworthy.

(Written by K. Sato and T. Fukui)