Investigating Δ and Ω Baryons as Meson–Baryon Bound States in Lattice Quantum Chromodynamics
© The Physical Society of Japan
This article is on
Lattice quantum chromodynamics (QCD) studies on decuplet baryons as meson–baryon bound states in the HAL QCD method
(PTEP Editors' Choice)
Prog. Theor. Exp. Phys.
2023,
043B05
(2023)
.
We investigate Δ and Ω baryons as meson–baryon bound states in lattice quantum chromodynamics and show that their difference results from the kinematic structure of the two meson–baryon systems, and not their interaction.
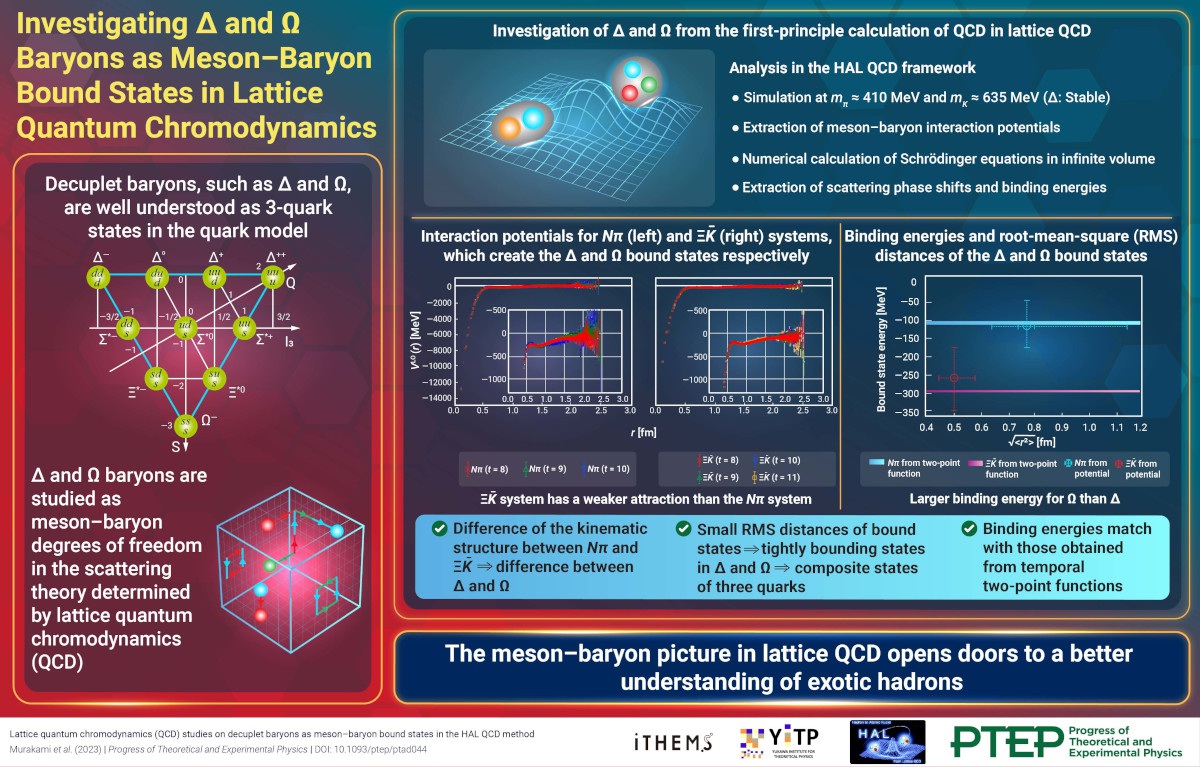
The quark model, as we know, provides a classification scheme for hadrons, composite subatomic particles made of two or more quarks held together by the strong interaction. While most hadrons are well understood in terms of the quark model, some like the so-called “exotic hadrons” are an exception. These hadrons appear as resonances arising from nonperturbative quantum chromodynamic (QCD) interactions. To understand their properties and internal structure, theoretical studies using first-principles lattice QCD are, therefore, necessary.
In this study, as a preliminary step towards understanding exotic hadrons in lattice QCD, we investigated decuplet baryons (such as Δ and Ω). While they are well described as 3-quark states, we studied them as meson–baryon degrees of freedom in the scattering theory determined by the lattice QCD calculation. Our method has the advantage that it can be applied to the exotic hadrons. In particular, we focused on why Ω appeared as a stable particle below the ΞK threshold while Δ becomes a resonance from the meson–baryon picture. To reduce the numerical cost, we used heavy quark masses, where Δ and Ω are stable particles. In this case, the difference of the decay properties between the two baryons is encoded in the inequality mN+ mπ − mΔ < mΞ+mK − mΩ.
We analyzed Δ and Ω baryons in the HAL QCD method, which helped us extract the meson–baryon interaction potentials directly in lattice QCD. In turn, we obtained the scattering amplitudes from potentials by solving the Schrödinger equations in an infinite volume. This enabled us to study Δ and Ω as bound states of Nπ and ΞK systems.
In our calculations, the binding energies determine the masses of Δ and Ω baryons, while the root-mean-square distances of the bound states qualitatively provides an estimate of the size of these baryons.
Upon extracting the interaction potentials, we found that the ΞK system has a weaker attraction compared to the Nπ system. However, the binding energy was larger for Ω than for the Δ baryon, manifesting in the form of the inequality mN + mπ − mΔ < mΞ+ mK− mΩ. Additionally, we discovered that a larger reduced mass in a ΞK bound state results in a smaller spatial size of the state. These results suggest that the difference between the two baryons is mainly due to the difference in the kinematic structure of the two meson–baryon systems rather than their interactions.
The calculations further revealed that the root-mean-squared distances of the bound states were small. This finding indicates tight binding states in Δ and Ω, where the two hadrons could essentially be considered as composite states of three quarks.
Our analyses of binding energies agreed with the results obtained from temporal two-point functions, indicating that our calculation method works well.
In summary, these findings shed light on the internal structure and properties of two decuplet baryons, opening doors to a better understanding of hadron spectroscopy including exotic hadrons, hadron interactions and, ultimately, the mysteries of our universe.
Lattice quantum chromodynamics (QCD) studies on decuplet baryons as meson–baryon bound states in the HAL QCD method
(PTEP Editors' Choice)
Prog. Theor. Exp. Phys.
2023,
043B05
(2023)
.
Share this topic
Fields
Related Articles
-
Understanding Global Inconsistency and ’t Hooft Anomaly Using Simple Quantum Mechanics
Theoretical Particle Physics
2025-2-21
This study explores the implications of global inconsistency and ’t Hooft anomalies for analyzing quantum field theories, using simple quantum mechanical models to provide valuable insights into these complex phenomena.
-
Understanding Non-Invertible Symmetries in Higher Dimensions Using Topological Defects
Theoretical Particle Physics
2024-9-27
By constructing Kramers-Wannier-Wegner duality and Z2 duality defects and deriving their crossing relations, this study presents the first examples of codimension one non-invertible symmetries in four-dimensional quantum field theories.
-
Quantum Mechanics of One-Dimensional Three-Body Contact Interactions
Mathematical methods, classical and quantum physics, relativity, gravitation, numerical simulation, computational modeling
Theoretical Particle Physics
2024-2-13
The quantum mechanical description of topologically nontrivial three-body contact interactions in one dimension is not well understood. This study explores the Hamiltonian description of these interactions using the path-integral formalism.
-
Investigating Unitarity Violation of Lee–Wick’s Complex Ghost with Quantum Field Theory
Theoretical Particle Physics
2024-1-19
Theories with fourth-order derivatives like Lee–Wick’s quantum electrodynamics model or quadratic gravity result in complex ghosts above a definite energy threshold that violate unitarity.
-
Novel Insights Into Bulk Reconstruction in the Anti-de Sitter/Conformal Field Theory Correspondence
Theoretical Particle Physics
2023-6-1
Bulk reconstruction in anti-de Sitter/conformal field theory is fundamental to our understanding of quantum gravity. We show that contrary to popular belief, bulk reconstruction is rather simple and intuitive.




