View larger image

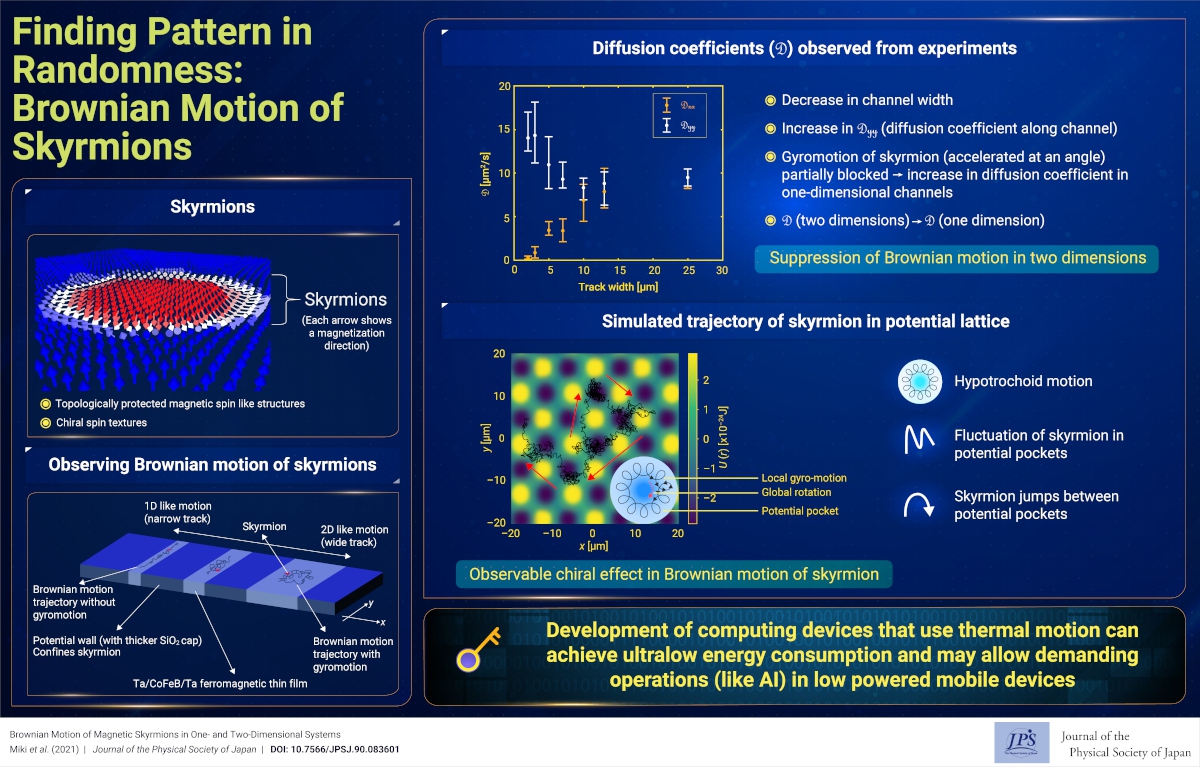
Magnetic skyrmions exhibit unique dynamical properties. However, it is unclear whether the Brownian motion of skyrmions exhibits chiral properties in thermal equilibrium derived from the Magnus force. For example, when a skyrmion is driven by an electric current, the driving force is parallel to the current. However, its trajectory is bent by the Magnus force, which is proportional to the skyrmion number. Consequently, the skyrmion Hall effect is observed. Such chiral motion of skyrmions has been observed in the presence of a driving force. However, whether the Brownian motion of skyrmions exhibits chiral properties in thermal equilibrium remains unclear. (Note that the chiral property in this study is not that of skyrmions but of their motion.) In this study, we show that chiral properties play an essential role in the diffusion of skyrmions by performing the following two experiments:

1. Diffusion of skyrmions in one-dimensional and two-dimensional channels

   The diffusion coefficient of skyrmions in one-dimensional narrow channels is a factor of 1.3–1.9 larger than that in two-dimensional films. This is because the confinement of skyrmions in one-dimensional channels suppresses their gyration.
   
   

2. Observation of angular momentum of diffusion in two-dimensional systems

   We evaluated chiral properties by analyzing the diffusion of skyrmions using the spontaneous velocity–position correlation functions, i.e., <v(t)・x(t)> and <(v(t) × x(t))_z> [1], and obtained the finite value of <(v(t)×x(t))_z> as a thermal average. This result indicates the existence of the off-diagonal term of the diffusion coefficient of the skyrmions' motion. In addition, the sign of the observed <(v(t)×x(t))_z> is opposite to the sign of the simple theoretical prediction, i.e., the direction of rotation is opposite to that predicted by theory. This can be explained by considering the shallow potential fluctuation in the films. The orbit of skyrmions captured by the harmonic potential was a hypotrochoid, a combination of two circular motions, with thermal fluctuation. The small, high-frequency circular motion was the intrinsic gyration of skyrmions. In contrast, a low-frequency orbit that rotated along the edge of the potential exhibited reverse rotation. Because the camera's frame rate was set at 30 ms⁻¹ in the experiments, only the global rotation in the direction opposite to that of the local gyration was observed. The observation of the intrinsic gyration and mass of skyrmions under Brownian motion should be examined in the future [2].

(Written by S. Miki on behalf of all authors)

References

[1] Y. Suzuki, S. Miki, Y. Imai, and E. Tamura, Phys. Lett. A, 413, 127603 (2021), and references therein.

[2] I. Makhfudz, B. Krüger, and O. Tchernyshyov, Phys. Rev. Lett. 109, 217201 (2012).