Evaluation of the Exchange Stiffness Constants of Itinerant Magnets from the First-Principles Calculations
© The Physical Society of Japan
This article is on
Evaluation of the Exchange Stiffness Constants of Itinerant Magnets at Finite Temperatures from the First-Principles Calculations
(JPSJ Editors' Choice)
J. Phys. Soc. Jpn.
93,
054705
(2024)
.
Using first-principles calculations, we evaluated the exchange stiffness constants of ferromagnetic metals at finite temperatures. The constants can be used as parameters in the Landau–Lifshitz–Gilbert equation.
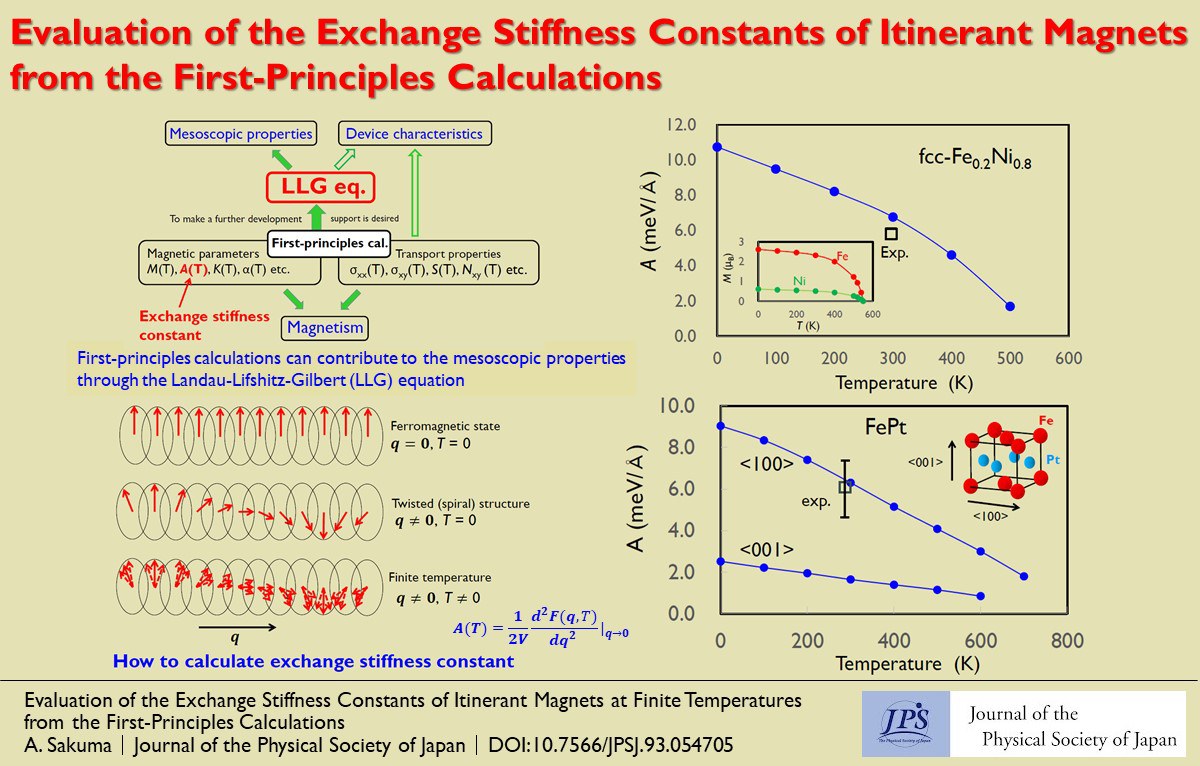
First-principles calculations based on electronic theory are powerful method for quantitatively representing the individuality of materials without the need for adjustable parameters. However, most industrial materials are far from perfect crystals and exhibit many defects such as vacancies, grain boundaries, and strains. Currently, it is difficult to describe the properties of these practical materials solely via first-principles calculations, and coarse graining based on the stiffness or characteristic length of order parameters is required. In such scale regions, the equations, such as the Landau-Lifshitz-Gilbert (LLG) equation for magnetism, are effective. This equation enables visualization of the macroscopic behavior of the order parameters (magnetization) in complex environments. Based on this perspective, the role of the first-principles calculations is to provide the parameters (magnetic constants) constituting the LLG equation.
The magnetic constants that constitute the LLG equation include the exchange stiffness constant (𝐴), magnetic anisotropy constant (K), and Gilbert damping constant (α). The issue arises because the interest in material properties predominantly focuses on room temperature or higher temperature regimes. Hence, the challenge involves determining the magnetic constants at finite temperatures from a microscopic standpoint. With respect to general metallic ferromagnets, functional integral methods have been powerful prescriptions for the finite temperature magnetism.
In this study, we focus on the first-principles calculations of the exchange stiffness constant 𝐴 at finite temperatures based on the functional integral method. The exchange stiffness constant is a measure of the rigidity (or hardness) of regular spin arrangements (such as ferromagnetic alignments) arising from the exchange interaction between spins. To evaluate 𝐴(𝑇), a spiral magnetic structure characterized by wavevector 𝑞 is generated in the presence of spin fluctuations. Subsequently, we can obtain 𝐴(𝑇) from the increase in free energy.
Specifically, we calculate 𝐴(𝑇) of Permalloy (Fe0.2Ni0.8) and L10-type FePt. For Fe0.2Ni0.8, the calculated magnetic moment is approximately 1 Bohr magneton (µB) at 𝑇 = 300 K, closely reproducing the measured values. The Curie temperature is approximately 550 K, slightly lower than the measured value (670 K). The obtained 𝐴(𝑇) falls within the range of values empirically used in the LLG equation (several meV/Å to 10 meV/Å), and 𝐴(𝑇) at room temperature appears to match well with the measured values. Additionally, 𝐴(𝑇) of FePt shows similar temperature dependence, aligning well with room temperature measurements. The results highlight the structure’s anisotropy, with significant directional dependence in 𝐴(𝑇).
Written by A. Sakuma
Evaluation of the Exchange Stiffness Constants of Itinerant Magnets at Finite Temperatures from the First-Principles Calculations
(JPSJ Editors' Choice)
J. Phys. Soc. Jpn.
93,
054705
(2024)
.
Share this topic
Fields
Related Articles
-
Exploring the Vibrant Interplay of Machine Learning and Physics
Cross-disciplinary physics and related areas of science and technology
Electron states in condensed matter
Elementary particles, fields, and strings
Mathematical methods, classical and quantum physics, relativity, gravitation, numerical simulation, computational modeling
Statistical physics and thermodynamics
Superconductivity
2025-3-13
This Journal of the Physical Society of Japan Special Topics edition explores how physics and machine learning complement each other and can solve unresolved problems in physics.
-
Excitonic Insulators: Challenges in Realizing a Theoretically Predicted State of Matter
Electron states in condensed matter
Electronic transport in condensed matter
2025-3-3
The realization of an excitonic insulator can help in the establishment of a new electronic state in condensed matter physics, one that has the potential to exhibit novel electric, magnetic, and optical responses beyond those of conventional materials.
-
Bayesian Insights into X-ray Laue Oscillations: Quantitative Surface Roughness and Noise Modeling
Measurement, instrumentation, and techniques
Structure and mechanical and thermal properties in condensed matter
2025-2-14
This study adopts Bayesian inference using the replica exchange Monte Carlo method to accurately estimate thin-film properties from X-ray Laue oscillation data, enabling quantitative analysis and appropriate noise modeling.
-
Hyperuniform and Multifractal States in Bosonic Quasicrystalline Systems
Statistical physics and thermodynamics
Structure and mechanical and thermal properties in condensed matter
2025-2-10
Quantum states can be categorized as hyperuniform or multifractal based on electronic characteristics. This study demonstrates that bosonic quasicrystalline systems exhibit hyperuniform or multifractal quantum states.
-
Spin-Spin Interaction Mediated by Rotational Lattice Vibrations
Magnetic properties in condensed matter
Structure and mechanical and thermal properties in condensed matter
2025-1-24
This study predicts the presence of spin-spin interactions mediated by the angular momentum of lattice vibrations, which can be long-range.




