Giant Vortices of Fish: Mechanisms and Patterns
© The Physical Society of Japan
This article is on
Emergence of a Giant Rotating Cluster of Fish in Three Dimensions by Local Interactions
(JPSJ Editors' Choice)
J. Phys. Soc. Jpn.
91,
064806
(2022)
.
The origin of giant rotating clusters of fish is revealed by a new agent-based model that incorporates the topological nature of interactions and the escape response characteristic to fish.
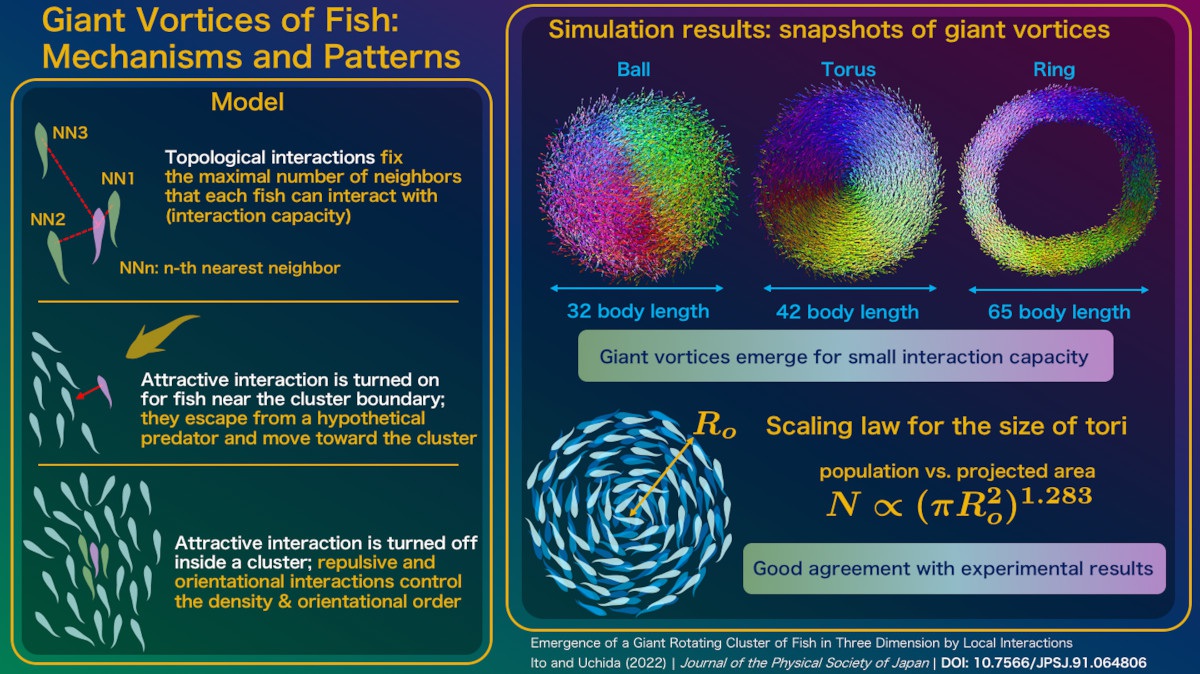
Fish schools form rotating balls, tori, and rings among other collective patterns.These vortex patterns are formed without inherent chirality of individual fish and are therefore considered as an example of spontaneous symmetry breaking due to interactions between self-propelling elements.
Origins of the vortex patterns are previously sought in potential-based attractive interactions, anisotropic interactions via a viewing angle, and/or long-range hydrodynamic interactions. However, several questions have remained on the formation of giant vortices consisting of tens of thousands of fish. For example, previous models require a large portion of fish in a school to interact with each other to form vortex patterns. However, in a high-density school of fish, visual and hydrodynamic interactions are screened, and thousands of fish simultaneously interacting with each other is unlikely. Furthermore, the dependence of the vortex size on the number of fish, which is known to obey a power law in schools of sardines and mackerels, has not been theoretically explained. Finally, three-dimensional rotating balls (known as "bait-balls") have not been reproduced in previous numerical studies.
In the present study, the above-mentioned problems are solved by a new model that incorporates the topological nature of interactions; fish are assumed to interact with up to a certain number of neighbors, which is called the interaction capacity. In addition, the attractive interactions are autonomously controlled such that they are turned on only when the fish has a small number of neighbors in the interaction range. This implies that fish near the cluster boundary are attracted by the cluster, which mimics the escape response characteristic to fish (“fast-start”). The model yields a rich phase diagram of collective patterns consisting of rotating balls, tori, rings, polarized schools, and randomly oriented swarms. The vortex patterns are obtained over a wide range of attraction strength when the interaction capacity is equal to or less than three. The projected area A of a torus scales with the number of fish N according to the power law N∝AV with the exponent ν = 1.283±0.004, which is close to the experimental value of 1.329. (Note that the values lie between 1 for a uniform disk and 3/2 for a uniform sphere.) Each fish in a torus exhibits random walk in the radial direction, instead of rigid-body rotation as predicted by non-topological models. These results indicate that the topological interactions enhance fluctuations in individual motion that trigger spontaneous breaking of the global rotational symmetry.
(Written by S. Ito and N. Uchida)
Emergence of a Giant Rotating Cluster of Fish in Three Dimensions by Local Interactions
(JPSJ Editors' Choice)
J. Phys. Soc. Jpn.
91,
064806
(2022)
.
Share this topic
Fields
Related Articles
-
Qualitative Changes in Kinetic Pathways Driven by Hydrodynamic Interactions in Dense Colloidal Suspensions
Cross-disciplinary physics and related areas of science and technology
Statistical physics and thermodynamics
Structure and mechanical and thermal properties in condensed matter
2025-4-18
Even in dense colloidal suspensions, where long-range hydrodynamic interactions are screened, near-field hydrodynamic interactions qualitatively influence the selection of kinetic pathways.
-
Exploring the Vibrant Interplay of Machine Learning and Physics
Cross-disciplinary physics and related areas of science and technology
Electron states in condensed matter
Elementary particles, fields, and strings
Mathematical methods, classical and quantum physics, relativity, gravitation, numerical simulation, computational modeling
Statistical physics and thermodynamics
Superconductivity
2025-3-13
This Journal of the Physical Society of Japan Special Topics edition explores how physics and machine learning complement each other and can solve unresolved problems in physics.
-
Exploring Materials without Data Exposure: A Bayesian Optimizer using Secure Computation
Cross-disciplinary physics and related areas of science and technology
Measurement, instrumentation, and techniques
2025-2-6
Secure computation allows the manipulation of material data without exposing them, thereby offering an alternative to traditional open/closed data management. We recently reported the development of an application that performs Bayesian optimization using secure computation.
-
Triangular Lattice Magnet GdGa2: Spin Cycloids and Skyrmions
Cross-disciplinary physics and related areas of science and technology
Electronic transport in condensed matter
Magnetic properties in condensed matter
2025-2-3
Careful measurements were conducted on the hexagonal magnet GdGa2 to reveal the experimental signatures of ultrasmall spin cycloids and of a potential Néel-type skyrmion lattice phase induced by a magnetic field.
-
Unlocking Secrets of Novel Charge-Orbital States in Transition-Metal Compounds
Cross-disciplinary physics and related areas of science and technology
Electron states in condensed matter
Electronic structure and electrical properties of surfaces and nanostructures
Magnetic properties in condensed matter
Structure and mechanical and thermal properties in condensed matter
2025-1-6
A new Special Topics edition of the Journal of the Physical Society of Japan features articles exploring special transition-metal compounds that exhibit novel charge-orbital states.




